13.2.3 & 13.2.4 Nuclear Physics

13.2.3 Describe one piece of evidence for the existence of nuclear energy levels.

For example, alpha (α) particles produced by the decay of a nucleus have discrete energies; gamma‑ray (γ-ray) spectra are discrete. Students should appreciate that the nucleus, like the atom, is a quantum system and, as such, has discrete energy levels.



The energies of both alpha and gamma are discrete (quantized) and have only certain values. Since these discrete energies come from the nucleus, it must follow that the nucleus has discrete nuclear energy levels. The graph below shows this property of alpha and is adapted from Heinemann HL Physics book by Chris Hamper.

13.2.4 Describe β+ decay, including the existence of the neutrino.

Students should know that β energy spectra are continuous, and that the neutrino was postulated to account for these spectra.


When energy calculations were done with beta emissions, scientists observed that the beta particles had less kinetic energy than expected. This opposed the law of conservation of energy (and in fact, scientists were so puzzled by this problem that they started to question the law of conservation of energy itself). In 1930, Wolfgang Pauli postulated that there was a virtually undetectable particle that therefore carried away this missing kinetic energy and momentum. This particle was named a 'neutrino'.


A neutrino is electrically neutral, has a very very small mass and travels at the speed of light.


In positron decay, a proton within the nucleus decays into a neutron and a positron (antimatter version of an electron--its antiparticle, when the two collide, they annihilate each other) is emitted. The equation for is is shown below (extracted from IB Physics Study Guides by Tim Kirk):

 Because of neutrinos and anti-neutrinos, beta emission forms a continuous spectrum. This is shown below (graph adapted from Heinemann HL Physics book by Chris Hamper).

13.2.1 & 13.2.2 Nuclear Physics

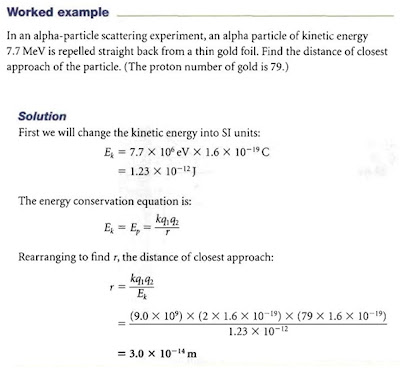
13.2.1 Explain how the radii of nuclei may be estimated from charged particle scattering experiments.

In charged particle scattering experiments, the particle that is repelled straight back will initially be momentarily at rest before it changes direction to travel straight back. Rutherford thus proposed that this fact can be used to estimate the size of the nucleus. This is because at that point at which the particle is at rest, its kinetic energy will be exactly balanced by its electrical potential energy due to the repulsive electrostatic force. 

13.2.2. Describe how the masses of nuclei may be determined using a Bainbridge mass spectrometer.

A magnetic field is used to deflect moving ions of a substance. If the ions have the same charge they will have the same velocity, v. The radius of their circular path however depends on the mass of the ion. A larger mass ion will travel in a larger circle. Therefore, the masses of nuclei may be determined using a Bainbridge mass spectrometer.

19.4
λ= 3.19 x 10^-7
f = v / λ
= 3 x 10^8 / 3.19 x 10^-7
= 9.4 x 10^14
φ + KE = hf
hf= 6.24 x 10^-18
KE = 5.86 x 10^-18 J
φ= 3.78 x 10^-19


Vs=KE=Vs x q
5.86 x 10^-18= Vs x 1.6 x 10^-19
Vs = 36.6 V


19.5
f = v / λ
f= 3x10^8 / 5 x 10^-7
=6 x 10^14
φ + KE = hf
6.63 x 10^-24 x 6 x 10^14 = φ + 2.4 x 10^-15
φ= 1.878 x 10^-19 J


KE now= 9 x 10^-19 J
hf= 1.878 x 10^-19 + 9x 10^-19
= 1.0878 x 10^-18
f= 1.64 x 10^15


λ = v/f
=3 x10^8 / 1.64 x 10^15
=1.83 x 10^-7 m

Quantam Physics - Q1,2,3,4

1) a) Drawn on one note
b) i) Planck's constant = gradient of line
m = change in y / change in x
m= 1.6 x 10^-19 / (7.4 x 10^14 - 4.9 x 10^14)
m= 6.4 x 10^-34 Js
Close to Planck's constant 6.6 x 10 ^-34

ii) 

KEmax = hf -φ
y = mx + c
( - because its a negative y-intercept)
Finding φ at 1.6 x 10^-19 and 7.4 x 10 ^14 Hz,
6.6 x 10 ^-34 x 7.4 x 10 ^14 = φ + 1.6 x 10^-19
φ= 3.284 x 10^-19
= 3.2 x 10^-19


b) If the frequency is lower than threshold frequency then KEmax will be more than hf and so subtracting KEmax from hf would yield a negative value. The work function cannot be a negative value.


2) a) Changes that occur in the micrometer when...
i) The intensity of incident light is increased (but frequency remains the same)
An increase in intensity will mean that more electrons will be liberated. As Q= IT, more electrons will mean more charge per unit time (Q/T increases) and as I = Q/T, I (current) will also increase. The microammeter will therefore detect an increase in current.
ii) The effect of increasing frequency will depend as to whether the starting frequency is the threshold frequency or not. If it is and the frequency is increased above the threshold frequency, the maximum energy of the electrons will  depend on the frequency of the incident light and will be higher as the frequency gets higher. If it is below threshold frequency and is increased to a value still below the threshold frequency, no photoelectrons will be emitted the current is still zero.


b) i)
 ii) Ek = 1.9 x 1.6 x 10^-19 = 3.04 x 10^-19 J
540 nm = 540 x 10^-9 m
v= fλ
3 x 10^8 = 540 x 10^-9 x f
f= 5.56 x 10^14 Hz
φ = hf - KEmax
φ= (6.6 x 10 ^-34 x 5.56x10^14) - 3.04 x 10^-19
φ= 6.3 x 10^-20




3) a) The de Brogile wavelength of a particle is the wavelength of a wave that determines the probability of a particle's position.

b) 
5.0 kV= 5.0 x 10^3 V
5.0 x 10^3 x 1.6 x 10^-19= 8 x 10^-16 J
Ek = 1/2 x m x v^2
8 x 10^-16 = 1/2 x 9.11 x 10^-31 x v^2
v= 4.2 x 10^7 ms^-1
p=mv
=9.11x 10^-31 x 4.2 x 10^7
p=3.8 x 10^-23


λ = h / p
λ= 6.6 x 10 ^-34 / 3.8 x 10^-23
λ= 1.7 x 10^-11


4) a) V= 75 V
Ek = 75 x 1.6 x 10^-19 = 1.2 x 10 ^-17
Ek = 1/2 x m x v^2
1.2 x 10 ^-17= 1/2 x 9.11 x 10^-31 x v^2
v= 5.1 x 10^6 ms^-1
p=mv
p= 9.11x10^-31 x 5.1 x 10^6
p= 4.67 x 10 ^-24
λ = h / p
λ = 6.6 x 10 ^-34 / 4.67 x 10 ^-24
λ = 1.4 x 10^10 m


b) The general shape of the graph mirrors the shape of a diffraction pattern as can be easily discerned from the maxima and minima. This graph therefore confirms de Brogile's hypothesis that electrons have both wave and particle properties.

12.3 Transmission of electrical power

12.3.1 Outline the reasons for power losses in transmission lines and real transformers.


Power is lost in transmission lines due to resistance (which causes the wires to heat up). Power losses also occur in real (or non-ideal) transformers due to the following reasons:

• Resistance of the windings of a transformer result in the transformer heating up.
• Eddy currents are unwanted currents induced in the iron core. The currents can be reduced by laminating the core into individually electrically insulated thin strips.
• Hysteresis losses causes the iron core to warm up due to the continued cycle of changes to its magnetism.
• Flux losses are caused by magnetic leakage. A transformer is only 100% efficient if all of the magnetic flux that is produced by the primary links with the secondary.

12.3.2 Explain the use of high-voltage step-up and step-down transformers in the transmission of electrical power.

If large amounts of power are being distributed, the current I will be very high. As the wires don't (and can't) have 0 resistance, they dissipate some power. Power dissipated is P = I^2 x R. If I is large then I^2 and therefore P will be  very large. Over a long distance, the power dissipated would be significant. The solution is to choose to transmit the power at a very high potential difference, such that only a small current needs to flow. A very high potential difference is much more efficient, but much more dangerous to the user. A step-up transformer is used to increase the voltage at the transmission stage and a step-down transformer is used to decrease it for use by the end user.


12.3.4 Suggest how extra-low-frequency electromagnetic fields, such as those created by electrical appliances and power lines, induce currents within a human body.


Electrical power lines carry alternating currents, which means they produce changing extra-low frequency electromagnetic fields. These changing fields are theoretically able to induce currents within any conductor nearby, including human bodies. The photons sent out by these electromagnetic fields therefore induce small currents in the human body, however these are too low to ionise.


12.3.5 Discuss some of the possible risks involved in living and working near high-voltage power lines.

• Electrical power lines on pylons are not insulated along their length and therefore are very dangerous if they become unattached from the pylons.
• There is also some statistical evidence to suggest that children who live near power lines are more likely to get leukemia. However, this relationship has not been properly proven and is not completely understood.

12.2 Alternating Current

12.2.1 Describe the emf induced in a coil rotating within a uniform magnetic field.

As the handle is turned, AB moves up through the field. As it cuts the field a current will be induced. Using Fleming's right hand rule --> direction of current is from A to B. Direction of motion of the right hand side (CD) is opposite so current is opposite. Result is a clockwise current through the resistor.


After turning half a revolution, side CD will now be moving up the field. Because of the slip rings, even though the current is still clockwise in the coil, it is anticlockwise in the resistor circuit.

12.2.2 Explain the operation of a basic alternating current (ac) generator.

A coil of wire rotates in the magnetic field due to an external force. As it rotates, the flux linkage of the coil changes with time and induces and e.m.f (refer to Faraday's law) causing current to flow.

Sides AB + CD will experience a force opposing the motion. (refer to Lenz's Law)

The work done rotating the coil generates electrical energy.

12.2.3 Describe the effect on the induced emf of changing the generator frequency.
If the speed of rotation is changed it will affect:


* Time period (distance between peaks)
* EMF (amplitude of peaks)


For example, if the speed is doubled therefore, the time period will be halved and the EMF (amplitude) will be doubled.

12.2.4 Discuss what is meant by the root mean squared (rms) value of an alternating current or voltage.

When the output of an a.c. generator is connected to a resistor, an alternating current will flow. A sinusoidal potential difference means a sinusoidal current. The graph shows that the average power dissipation is half the peak power dissipation for a sinusoidal current.

Thus the effective current through the resistor is (mean value of I^2)^0.5 and it is called the root mean square (rms) current. It is also know as the rating.


12.2.5 State the relation between peak and rms values for sinusoidal currents and voltages.

Mean value:



The rms value is the squareroot of this...

The current passing through the resistor will be proportional to the potential difference across it, so this will be sinusoidal, if the peak current is Io, the rms value will therefore be given by:

Questions
42. rms V =110 V
Vrms x V2 = 156 V


43. 4kW = 4000W , 220 V
P= VI, therefore I = P / V
I = 4000 / 220 = 18 A (to 2.s.f)


44. a) i) Calculating the angular velocity:

w= 2π x 50 = 100π rad s-1

ii) Calculating the maximum induced emf

BANw= Emax

100π x 500 x 5 x 10^-4 x 50 x 10^-3

= 3.9 V

iii) Calculating the rms emf 

= 2.8 V

b) Calculating the new Erms if the speed is reduced to 25 revolutions per second

Eo = 1.95 V

Erms= 1.95 / (2)^0.5 = 1.4 V

45. Calculate the resistance of a 1000 W bulb designed to operate at 220 V.


P = V^2 / R
R = V^2 / P
= 220^2 / 1000 = 48.4 ohms

Topic 12 Homework 04/01/2011

Syllabus Statements: 12.1.1 to 12.1.5

12.1.1 Describe the inducing of an emf by relative motion between a conductor and a magnetic field.

A conductor is full of free electrons. When it is moved through a magnetic field, a magnetic Force b (that can be found using Flemmings Left Hand rule) acts on the electrons and pushes the electrons to one side of the conductor. This creates a side with a negative charge and another side with a positive charge and thus creates a potential difference between the ends of the conductor. Due to this potential difference, an emf is induced. Force e acts equal but opposite to Force b and therefore the electrons stay in place (stop moving).

12.1.2 Derive the formula for the emf induced in a straight conductor moving in a magnetic field.

12.1.3 Define magnetic flux and magnetic flux linkage.

Magnetic flux: The product of magnetic field strength and a perpendicular area.

Magnetic flux linkage: The product of the no. of turns of a coil and magnetic flux.

12.1.4 Describe the production of an induced emf by a time-changing magnetic flux.

Changing the direction of the magnetic field has the same effect as moving the conductor in 12.1.1. As the magnetic field changes direction, it changes the direction of the force on the electrons and thus gives rise to an induced current.

12.1.5 State Faraday’s law and Lenz’s law.

Faraday's law: The induced emf is equal to the rate of change of flux.

Lenz's law: The direction of the induced current is such that it will oppose the change producing it.

Homework Questions

Pages 211 & 213

Homework Page 1

Homework Page 2